Home | Up One Level | Previous | Next
Table of Contents | Previous Section | Next Section
ELECTRODYNAMIC AND ELECTROMAGNETIC ACTIONS
§6. –ACTION OF A CLOSED CURRENT ON A NEUTRAL
CURRENT ELEMENT
WALTER RITZ
Translated from Recherches
critiques sur l'Ėlectrodynamique Générale,
Annales de Chimie et de Physique, Vol. 13, p. 145, 1908.
Annales 233
(Oeuvres 390)
Let 
be the positive and
negative charges of two elements in two wires, one situated at x', y',
z', the other at x,
y, z. By hypothesis, the total
charges 
are very small in
relation to
,… The force exerted by ds' on ds is the sum of actions of
and
on
and
. The speeds of the positive
ions and of the substance are V, V';
those of the negative ions, v, v'; the
relative speeds V-v=η, V'-v'= η', and
consequently, J, J' have the direction (Oeuvres 391)ds (dx, dy, dz)
and ds'
(dx',
dy', dz'); thus we will
have
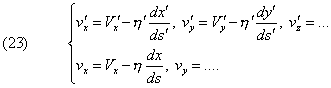
Let us put these values in expression (13) of Fx,
and do the indicated sum. The term 
will have a
coefficient of
;
this is the electrostatic action.
The terms in 
will be, to a
factor of about ds ds',
the non-written terms being deduced from these by circular permutation
of x y z. The expression may be written
When the resulting charges E, E'
are nil, only the last one subsist. In the other cases, 
are very small, and as the whole must be multiplied by 1/c2,
one can see that the first term is absolutely negligible compared to the
electrostatic term. The second term corresponds to a very weak action of a
neutral current on an electrostatic charge in motion, the third one to the
action of such a charge on a current or a magnet (Rowland effect). These two
effects are of the same order of magnitude, and manifest themselves only
(Oeuvres 392) in very delicate experiments and when V or V'
are considerable. If the electrostatic term itself is weak, as it is in
general, they are absolutely negligible. We will discuss the Rowland effect
later on.
The squared terms are also very small in relation
to
, and only this last term remains:
Likewise, the terms in 
and 
perceptibly give only
a result proportional to JJ', and we will have, for the
ensemble of terms dependent on
the speeds,
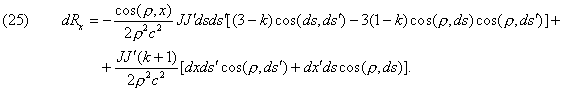
According to Lorentz’s formula (20), the action of ds'
on ds is, by
virtue of analogous reasoning, given by
the terms in E, E' and the squared terms again being
negligible. As for the term containing the accelerations, which is the same in
both theories, it is again multiplied by 
, and is consequently negligible, unless the accelerations
are very large, which isn’t the case under experimental conditions where one
can observe the electrodynamic or electromagnetic actions.
I say that the resultant of the action of a
closed circuit ds' on
the element ds is the same following (25) and (26). In fact,
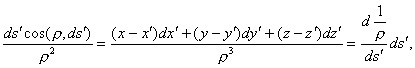
(Oeuvres 393) because
The term in dx ds' cos(ρ,ds')
is consequently a total differential in relation to s'; its
integral is nil for
a closed contour. Likewise
and the integration by parts along s' will transform
this term into
so that it comes, for the
sought resulting action experienced by ds,
This is exactly what the integration of (26) would
give. The constant k disappears from the result. Besides, the latter is
independent of the motion of the wires or of their deformation, provided that J
and J' stay constant, that the element of current J ds
be neutral, and that J' be closed and neutral. The
rotational actions of the currents and the magnets are thus explained in a
classical manner.
For k= -1, the action of two elements
of neutral current would be exactly given by the formula of Ampère; this
formula may thus be admitted even today.
When there is a question about a body of two or
three dimensions, of which one is traversed by a neutral current decomposable
into closed filaments, the latter acts on the other elements of volume in
accordance to the formula obtained; but, furthermore, the currents themselves
slightly change their direction, as we shall see in the following (Oeuvres 394)
section: this is the Hall effect. But this effect is minimal, and may be
disregarded here.
As always, the effects of magnetism will be
obtained by replacing magnets by molecular closed neutral currents; here again,
there will thus be no difference between the theories.
Table of Contents | Previous Section | Next Section