Table of Contents | Previous Section | Next Section
§ 4. -- ENERGY
WALTER RITZ
WALTER RITZ
Translated (1980) from
Recherches critiques sur l'Électrodynamique Générale,
Annales de Chimie et de Physique, Vol. 13, p. 145, 1908.
Annales 172 (Oeuvres 340)
Poynting has since shown that this results from the general equations, that
for all closed surfaces σ we have
is the surface component in the direction of the normal external
radial vector
We will set aside this metaphysical question to study the indetermination that this mode of representation suffers. (Oeuvres 341) The electrostatic energy of a system is
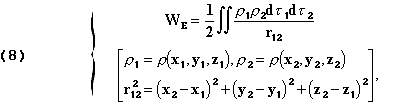
the two integrations being extended over all space wherever electric charges are found.
It is evident that this expression can be put in the form of an extended integral throughout all space in an infinite number of ways and the one Maxwell has chosen is, from the point of view of his system, particularly simple. But we can show others which will have for example,
(1)See W. Wein, Annalen der Physik u. Chemie, 2nd series,
volume XLV, 1892, p. 684.
the advantage of drawing closer to the forms used in the theory of elastic
bodies. Lets therefore introduce, instead of the electric force E in
x,y,z which is
for which
Now, if
were the
components of displacements for an elastic body, the energy of this
latter vector would be, as we know
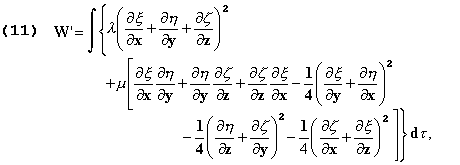
λ and μ being constants of elasticity of the body, or, in adding the
(Oeuvres 342) zero terms
, . . . and transforming
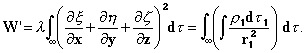
For electricity, supposedly being firmly attached to small solid bodies
(ions) 
, we have
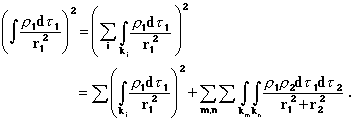
The first term doesn't depend on the mutual positions of the bodies. Its integral with regard to
extended over all space will be a constant. In the double summation the
variables 
assume the
gamut of relative values for a given combination of different ions
. We therefore never
have 
which allows the
transposition of the order of integration and we write
To evaluate the integral taken with respect to
let's introduce polar coordinates
with
as the polar origin, the line joining
and
as the polar axis. Let
be the distance between these two points. We have to calculate
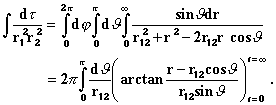
(Oeuvres 343)
The principal values (included between
and
) are
for
, and
for
.
At last we find
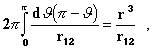
from which
We conclude finally, in choosing
λ = 1/2π3,
that with the hypotheses made and with the units introduced, the
elastic energy W' will, having a nearly constant summation, be
equal to the electrical energy
given by (8), and that we have in particular the formula
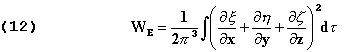
which is irreducible to Maxwell's formula, and gives a completely
different distribution for the energy. It would be easy to obtain, by
starting in this manner, a similar expression for magnetic energy and,
consequently, for currents; and we see that, even in insisting on the
simplest of formulas, the localization of energy is still an indeterminant
problem.
It is the same for the flux of energy.(1) We can always modify the motion
of the flowing energy in an arbitrary way by adding to Poynting's vector
another vector (u,v,w) obliged only to satisfy the equation for
incompressible fluids.
from which
Poynting's theorem, being a consequence of the general equations, doesn't add anything to them. The localization of energy
* This is the second equation numbered "11."
(1) See the article of M. Voss in Encyklop. d. math. Wissenschaften,
vol. IV, art. 1, 1901,
p. 111-114.
must therefore be attributed to a number of logically useless (and
maybe harmful at times) conceptions in the theory.
The primary source of the conservation of energy theorem has been, and will continue to be, the experimental impossibility of perpetual motion, an impossibility that must exist whatever our ideas may be on the portions of energy that the ether is obliged to supply in the absence of material bodies. The energy theorem, in its classical form
explained this impossibility. Poynting's theorem, in demanding only the possibility of the transformation of an integral of volume (already partly arbitrary) to an integral of surface, expresses a lot less. Far from giving an account for this impossibility, it readily allows the creation of a perpetual motion. This is to say that, as long as we have not introduced the hypothesis of retarded potentials, a continuous portion of the energy, from converging waves coming from infinity, remains just as possible as the lost energy that we observe in reality. If an engine could perpetually draw energy from ether solely, independently of the presence of material bodies, it could have perpetual motion. We know therefore, that in adopting the formula for retarded potentials, we must show(1) that an accelerated particle loses energy and undergoes, as a result, a reaction proportional to the derivative of its acceleration. We only have to change the sign of c to pass to the hypothesis of convergent waves. We see then that the sign of the radiant vector changes also, and
(1) LORENTZ, Elektronentheorie, p. 186 - LARMOR,
Aether and Matter, Chap. XIV.
the new hypothesis will lead, for example in the case of a vibrating
particle, to a continuous increase in the amplitude with time, and more
generally to an increase of energy of the system.
Poynting's theorem expresses the law of energy only when we replace
the fields with their representations based on retarded potentials, a
restriction which removes a lot of its elegance and scope.
If we begin from a state in which radiation is sensibly nil and where
the energy 
can be converted into work, and we arrive at an (Oeuvres 345)
analogous state (energy 
), the system supposedly isolated from the action of external
bodies, we will have, according to Lorentz's theory, (which supposes
the admissibility of the formula for potentials)
the equality taking place only if radiation is continuously nil. The impossibility of perpetual motion also produces, in an essentially irreversible system, only the inequality. The energy can never increase. There is a parallelism in this relation with the law of entropy. In fact, electromagnetic energy is not conserved in general. This is to say that W= const. doesn't exist. We save the law of conservation of energy by attributing to the ether the lost quantity, and this procedure has decidedly great advantages, especially when we can completely recover the energy lost by the system by means of bodies that don't sensibly exert action on it, like the black bodies of optics. But with this energy not producing, in this hypothetical setting, any modifications which would be perceptible to our senses, we can ask ourselves if, under these conditions, would it not be possible to save likewise all other similar laws, as we have effectively done for electromagnetic momentum.
In the most general case of electromagnetic radiation, conservation of energy is no longer a law, but a convention. This is a fairly frequent development in the domain of physical truths, as stated by Poincaré(1).
(1) POINCARÉ, Science et Hypothese, Paris, 1901. He says, in
closing, that the statement "the energy of the whole world conserves
itself" does not make any sense, save for a space having a positive
curvature.
Copyright © 1980, 2000 Robert S. Fritzius
This section of the translation is dedicated to the memory of
Aubrey Hunter Fritzius 1940-1982.
Installed November 13, 2000
Table of Contents |
Previous Section |
Top |
Next Section
Previous |
Up one level |
Next
Copyright © 1980, 2000 Robert S. Fritzius
This section of the translation is dedicated to the memory of
Aubrey Hunter Fritzius 1940-1982.
Installed November 13, 2000
Table of Contents |
Previous Section |
Top |
Next Section
Previous |
Up one level |
Next